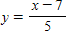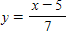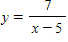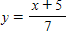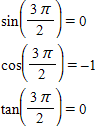Solve the problem.The annual population density of a species of insect after n years is modeled by a sequence. Suppose that the initial density of insects is 606 with r = 0.8. Write a recursive sequence that describes this data, where an denotes the insect density during year n.Find the terms a1 , a2 , a3 , ...., a5 . Round to two decimal places, if necessary.
A. a1 = 606, a2 = 606, a3 = 606, a4 = 606, a5 = 606
B. a1 = 484.80, a2 = 387.84, a3 = 310.27, a4 = 248.22, a5 = 198.57
C. a1 = 606 , a2 = 484.80, a3 = 387.84, a4 = 310.27, a5 = 248.22
D. a1 = 606, a2 = 1090.80, a3 = 1963.44, a4 = 3534.19, a5 = 6361.55
Answer: C
You might also like to view...
Solve the problem.What principal invested at 6%, compounded continuously for 3 years, will yield $1500? Round the answer to two decimal places.
Fill in the blank(s) with the appropriate word(s).
Decide whether or not the functions are inverses of each other.
A. No B. Yes
Find the inverse of the one-to-one function.
?
?
A. 
B. 
C. 
D. 
E. none of the above
Evaluate (if possible) the sine, cosine, and tangent of the real number.
?
?
A. 
corresponds to the point 
.
B.
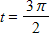corresponds to the point 
.?
?
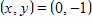C.
corresponds to the point 
.?
?
D.
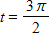corresponds to the point 
.?
?
E. Not possible