Solve the problem.A rectangular box with volume 433 cubic feet is built with a square base and top. The cost is $1.50 per square foot for the top and the bottom and $2.00 per square foot for the sides. Let x represent the length of a side of the base. Express the cost the box as a function of x.
A. C(x) = 2x2 + 
B. C(x) = 3x2 + 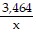
C. C(x) = 4x + 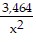
D. C(x) = 3x2 + 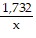
Answer: B
Mathematics
You might also like to view...
Find the derivative of y with respect to x.y = -cos-1 
A. 
B. 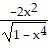
C. 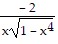
D. 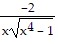
Mathematics
Find the discount and proceeds on the simple discount note.Face value: $47,300.00Discount rate: 9.75%Time (days): 100
A. Discount: $0.00 Proceeds: $47,300.00 B. Discount: $1,281.04 Proceeds: $46,018.96 C. Discount: $4,611.75 Proceeds: $42,688.25 D. Discount: $1,263.49 Proceeds: $46,036.51
Mathematics
Evaluate the numerical expression.324/5
A. 16 B. 256 C. 512 D. 128
Mathematics
Tell which signed number corresponds to the situation.$4304 out of debt
A. +4304 B. -4304
Mathematics