A club has seven members. Three are to be chosen to go as a group to a national meeting.
(a) How many distinct groups of three can be chosen?
(b) If the club contains four men and three women, how many distinct groups of three contain
two men and one woman?
(c) If the club contains four men and three women, how many distinct groups of three contain
at most two men?
(d) If the club contains four men and three women, how many distinct groups of three contain
at least one woman?
(e) If the club contains four men and three women, what is the probability that a distinct
group of three will contain at least one woman?
(f) If two members of the club refuse to travel together as part of the group (but each is
willing to go if the other does not), how many distinct groups of three can be chosen?
(g) If two members of the club insists on either traveling together or not going at all, How
many distinct groups of three can be chosen?


You might also like to view...
Write an equation in standard form using only integers for the line described.The line through (4, 4), parallel to y = - 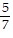 x + 1
x + 1
A. 5x - 7y = 48 B. 7x + 5y = -48 C. 5x + 7y = -48 D. 5x + 7y = 48
Provide an appropriate response.Under what conditions must the inequality symbol be reversed when solving an inequality?
What will be an ideal response?
Graph the linear function.f(x) = -4x + 6
A. 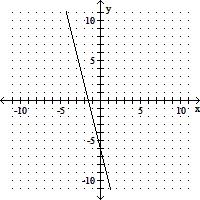
B. 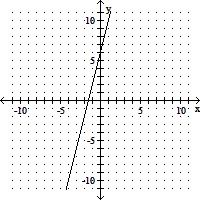
C. 
D. 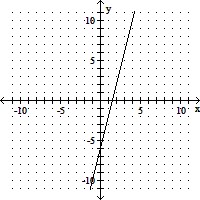
Solve the problem.The Paperback Trader is a book store that takes in used paperbacks for 20% of their cover price and sells them for 50% of their cover price. Pat brings in a stack of 17 paperback books to trade and gets $13.73 credit. Some of the books had a cover price of $5.98, the rest $2.98. She wants to get some Tom Clancy books having a cover price of $5.98. How many $5.98 books did she bring in and how many Clancy books can she get without paying any additional cash?
A. 6 $5.98 books, 4 Clancy books B. 6 $5.98 books, 2 Clancy books C. 6 $5.98 books, 5 Clancy books D. 11 $5.98 books, 2 Clancy books