Find all the second order partial derivatives of the given function.f(x, y) = cos xy2
A. fxx(x, y) = y2 sin xy2; fyy(x, y) = 2[2y2 cos (xy2) - sin (xy2)] ; fyx(x, y) = fxy(x, y) = 2y[y2 cos (xy2) - sin (xy2)]
B. fxx(x, y) = -y4 cos xy2; fyy(x, y) = - 2x[2xy2 cos (xy2) + sin (xy2)]; fyx(x, y) = fxy(x, y) = - 2y[xy2 cos (xy2) + sin (xy2)];
C. fxx(x, y) = - y2 sin xy2; fyy(x, y) = 2[ sin (xy2)- 2y2 cos (xy2)] ; fyx(x, y) = fxy(x, y) = 2y [sin (xy2)-y2 cos (xy2)]
D. fxx(x, y) = - y2 sin xy2; fyy(x, y) = 2y ; fyx(x, y) = fxy(x, y) = 2
; fyx(x, y) = fxy(x, y) = 2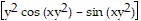
Answer: B
You might also like to view...
Evaluate or simplify the exponential expression.-43
A. 256 B. 64 C. -16 D. -64
Write the phrase as a mathematical expression. Use x as the variable.397 divided by some number
A. 397 + x
B. 397 - x
C. 397x
D. 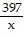
Graph the function by starting with the graph of the basic function and then using the techniques of shifting, compressing, stretching, and/or reflecting.f(x) = 


A. 
B. 
C. 
D. 
Solve the equation.50 = 7x - 6
A. 53 B. 8 C. 13 D. 49