Find the value of the objective function at each corner of the graphed region. What is the maximum value of the objective function? What is the minimum value of the objective function?Objective Function z = 5x + 6y
A. maximum value: 61; minimum value: 42
B. maximum value: 42; minimum value: 11
C. maximum value: 64; minimum value: 61
D. maximum value: 64; minimum value: 11
Answer: D
You might also like to view...
Solve the problem.Let V be in ?4, involving evaluation of polynomials at -4, -1, 0, 1, and 4, and view ?2 by applying the Gram-Schmidt process to the polynomials 1, t, and t2.
A. p2(t) = t2 + 6
B. p2(t) = t2 - 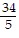
C. p2(t) = t2 + 
D. p2(t) = t2 - 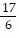
Evaluate the trigonometric function for the given value of t.sin t, t = 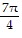
A. 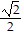
B. 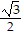
C. - 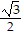
D. - 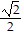
Use the product rule to simplify the radical.5
A. -85
B. 25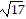
C. 5
D. 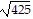
Solve the problem.A radioactive substance has a decay rate of 1.9% per minute. Of an initial amount of 1000 g of the substance, how much will remain after 80 minutes?
A. 218.7 g B. 196.8 g C. 295.3 g D. 245 g