Finish the proof.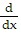 cos 2x = -2 sin 2x. (Use the double-angle identity.)
cos 2x = -2 sin 2x. (Use the double-angle identity.)
A.  cos 2x =
cos 2x = 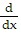 (cos2 x - sin2 x) =
(cos2 x - sin2 x) =  [(cos x)(cos x) - (sin x)(sin x)]
[(cos x)(cos x) - (sin x)(sin x)]
= (-sin x)(cos x) + (cos x)(-sin x) - (cos x)(sin x) + (sin x)(cos x) = -2 sin x cos x = -2 sin 2x
B. 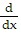 cos 2x =
cos 2x =  (cos2 x - sin2 x) =
(cos2 x - sin2 x) =  [(cos x)(cos x) - (sin x)(sin x)]
[(cos x)(cos x) - (sin x)(sin x)]
= (-sin x)(cos x) + (cos x)(-cos x) - (cos x)(sin x) - (sin x)(sin x)
= -2 sin x cos x - cos2 x - sin2 x = -2 sin 2x
C.  cos 2x =
cos 2x = 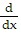 (cos2 x - sin2 x) =
(cos2 x - sin2 x) =  [(cos x)(cos x) - (sin x)(sin x)]
[(cos x)(cos x) - (sin x)(sin x)]
= (-sin x)(cos x) + (cos x)(-sin x) - (cos x)(sin x) - (sin x)(cos x) = -4 sin x cos x = -2 sin 2x
D.  cos 2x =
cos 2x = 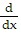 (cos2 x - sin2 x) =
(cos2 x - sin2 x) = 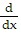 [(cos x)(cos x) - (sin x)(sin x)] = 2 cos x - 2 sin x
[(cos x)(cos x) - (sin x)(sin x)] = 2 cos x - 2 sin x
= -4 sin x cos x = -2 sin 2x
Answer: C
You might also like to view...
Evaluate the line integral along the curve C.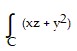 ds, C is the curve r(t) = (-8 - 2t)i + tj - 2tk , 0 ? t ? 1
ds, C is the curve r(t) = (-8 - 2t)i + tj - 2tk , 0 ? t ? 1
A. - 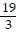
B. 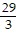
C. 29
D. - 19
Find the unknown number in the proportion. Round answer to the nearest hundredth when necessary. =
= 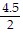
A. x = 6.75 B. x = 13.5 C. x = 22.5 D. x = 20.25
Find the slope-intercept form of the equation of a line that passes through the given points.(8, 10), (-3, 10)
A. y = 8
B. y = 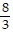 x
x
C. y = 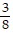 x
x
D. y = 10
Check the addition. If the answer is incorrect, find the correct answer.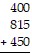 1655
1655
A. Correct B. Incorrect; should be 1765 C. Incorrect; should be 1665 D. Incorrect; should be 1565