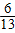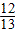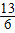Provide an appropriate response.With the function f(x) = logax, why can't x be less than 0?
What will be an ideal response?
Answers may vary. One possibility: The function is defined for all positive numbers a, 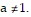 Since a positive number raised to any real-number exponent is a positive number, x must be positive, too. That begs the question of why a must be positive. Assume that a is a negative number. A negative number raised to certain powers (e.g., 1/2) would yield a non-real number.
Since a positive number raised to any real-number exponent is a positive number, x must be positive, too. That begs the question of why a must be positive. Assume that a is a negative number. A negative number raised to certain powers (e.g., 1/2) would yield a non-real number.
You might also like to view...
Solve the problem.V =  ?r3, where r is the radius, in centimeters. By approximately how much does the volume of a sphere increase when the radius is increased from 1.0 cm to 1.1 cm? (Use 3.14 for ?.)
?r3, where r is the radius, in centimeters. By approximately how much does the volume of a sphere increase when the radius is increased from 1.0 cm to 1.1 cm? (Use 3.14 for ?.)
A. 0.1 cm3 B. 1.5 cm3 C. 1.3 cm3 D. 1.1 cm3
Factor.343x6 + 8y6
A. (7x2 + 2y2)(49x4 + 4y4) B. (7x3 + 2y3)(49x3 - 14x3y3 + 4y3) C. (7x2 - 2y2)(49x4 + 14x2y2 + 4y4) D. (7x2 + 2y2)(49x4 - 14x2y2 + 4y4)
Divide the polynomials using polynomial long division.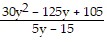
A. 5y + 15 B. 6y + 132 C. 6y + 7 D. 6y - 7
?Find the value of the constant a that makes the given function a probability density function on the stated interval.
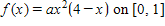
A. ?
B. ?
C. ?
D. ?
E. ?1