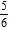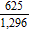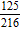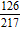Solve the problem.A 2000-L tank is initially filled with a copper sulfate solution with a concentration of 50 g/L. A copper sulfate solution with a concentration of 20 g/L flows into the tank at a rate of 15 L/min. The thoroughly mixed solution is drained from the tank at a rate of 15 L/min.(a) Write an initial value problem for the mass of the copper sulfate.(b) Solve the initial value problem.
A. (a) m'(t) = -0.007m + 300, m(0) = 100,000
(b) m = 60,000e-0.007t + 40,000
B. (a) m'(t) = -0.007m + 20, m(0) = 50
(b) m = -39,950e-0.007t + 40,000
C. (a) m'(t) = 0.008m + 300, m(0) = 50
(b) m = -39,950e0.008t + 600,000
D. (a) m'(t) = -133.333m + 300, m(0) = 100,000
(b) m = 60,000e-0.007t + 600,000
Answer: A
Mathematics
You might also like to view...
Add or subtract as indicated. Write the answer in simplest form.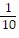 +
+ 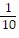 +
+ 
A. 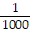
B. 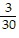
C. 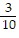
D. 
Mathematics
Find the solution to the system by addition (elimination) method. 2x + 4y = -14 8x + 2y = 42
A. (7, -7) B. (-7, 7) C. (-2, 7) D. (8, -8)
Mathematics
Use the square root property to solve the equation.(5x + 7)2 = 36
A.  ,
, 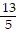
B. - 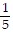 , 0
, 0
C. -  , -
, - 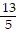
D. 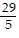
Mathematics
Simplify the expression.
A.
B.
C.
D.
E.
Mathematics