Prove that the equation is an identity.2 sin(x + y) cos(x - y) = sin 2x + sin 2y
What will be an ideal response?
2 sin(x + y) cos(x - y) = 2[sin x cos y + cos x sin y] [cos x cos y + sin x sin y]
= 2(sinx cos x cos2y + sin2x cos y sin y + cos2x sin y cos y + sin2y cos x sin x)
= 2[ sin x cos x(sin2y + cos2y) + sin y cos y (sin2x + cos2x) ]
= 2[sin x cos x ? 1 + sin y cos y ? 1] Using the Pythagorean identity
= 2 sin x cos x + 2 sin y cos y
= sin 2x + sin 2y Using the double-angle identity
You might also like to view...
A point is located five units below the x-axis and the coordinates of the point are equal. Find the coordinates of the point. ?
A. (-5, 5) B. (-5, 0) C. (5, -5) D. (-5, -5) E. (5, 5)
Plot the point in the rectangular coordinate system.(1, -5)
A.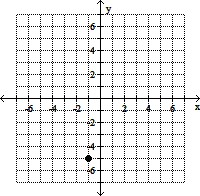 
B.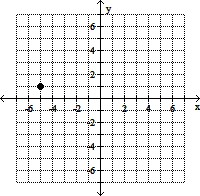 
C. 
D.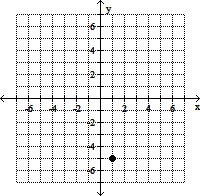 
Determine whether the given points are on the graph of the equation.y = x3; (-4, -64)
A. No B. Yes
Solve the problem.Find the polar coordinates of the point(s) of intersection of the given curves for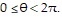 
r1 = 4, r2 = 4 + sin ?
A. (0, 0), (0, 4), (?, 4) B. (0, 4), (?, 4) C. (0, 0), (4, 0), (4, ?) D. (4, 0), (4, ?)