When satellites observe Earth, they can scan only part of Earth's surface. Some satellites have sensors that can measure the angle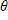 
shown in the figure. Let h represent the satellite's distance from Earth's surface and let r represent Earth's radius. Find the rate at which h is changing with respect to 
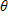when
src="https://sciemce.com/media/2/ppg__cognero__Section_2.3__media__46425330-7b24-499e-801e-3eb8d4032706.PNG" class="wirisformula" align="middle" style="vertical-align: middle;" data-wiris-created="true" varid="variable_id_field" variablename="impvar_8bacf3e1a4ae48a4a332f5ddf" /> (Assume 
miles.) Round your answer to the nearest unit.
?
?
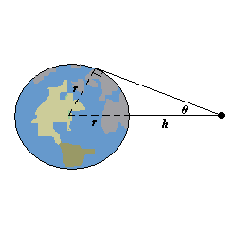
A. -2,642 mi/radian
B. -4,576 mi/radian
C. 4,576 mi/radian
D. -7,926 mi/radian
E. 2,642 mi/radian
Answer: A
Mathematics
src="https://sciemce.com/media/2/ppg__cognero__Section_2.3__media__46425330-7b24-499e-801e-3eb8d4032706.PNG" class="wirisformula" align="middle" style="vertical-align: middle;" data-wiris-created="true" varid="variable_id_field" variablename="impvar_8bacf3e1a4ae48a4a332f5ddf" /> (Assume miles.) Round your answer to the nearest unit.
?
?
A. -2,642 mi/radian
B. -4,576 mi/radian
C. 4,576 mi/radian
D. -7,926 mi/radian
E. 2,642 mi/radian
Answer: A
You might also like to view...
Use a graphing calculator to evaluate the series.
A. -11 B. 189 C. 273 D. 73
Divide and simplify. Write the answer as a whole or mixed number when necessary.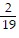
÷ 
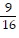
A.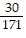 
B.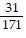 
C.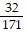 
D.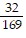 
Divide using synthetic division, and write a summary statement in fraction form.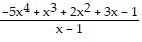
A. -5x3 + 6x2 - 4x - 1 B. -5x3 + 4x2 + 6x + 9 C. -5x3 - 4x2 - 2x + 1 D. -5x3 + 4x2 - 2x - 1
Describe the transformation that sends ?ABC to ?A'B'C' in Figure 1. Mark the relevant vectors, points, lines, etc. and give the coordinates for these objects as appropriate.
