Use mathematical induction to prove the following.0.25n < 0.25n-1
What will be an ideal response?
Answers may vary. One possibility:
Sn: 0.25n < 0.25n-1
S1: 0.251 < 0.251-1
Sk: 0.25k < 0.25k-1
Sk+1: 0.25k+1 < 0.25k
1. Basis step: Since 0.251-1 = 0.250 = 1 and 0.251 < 1, S1 is true.
2. Induction step: Let k be any natural number. Assume Sk. Deduce Sk+1.
0.25k < 0.25k-1
0.25k ? 0.25 < 0.25k-1 ? 0.25 Multiplying by 0.25, 0.25 > 0
0.25k+1 < 0.25(k-1)+1
0.25k+1 < 0.25k.
You might also like to view...
Divide.
A. 9 B. 19 C. 1.9 D. 0.9
Use algebraic and/or graphical methods to solve the inequality.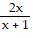 ?
? 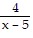
A. -1 < x ? 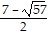 or 5 < x ?
or 5 < x ? 
B. -1 < x < 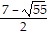 or 5 < x <
or 5 < x < 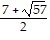
C. -1 < x ? 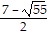 or 5 < x ?
or 5 < x ? 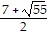
D. -1 < x ?  or 5 < x ?
or 5 < x ? 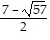
Solve the system by the elimination method. x + 4y = -4-5x + 5y = -5
A. {(0, -1)} B. ? C. {(1, -2)} D. {(1, 0)}
Factor completely, or state that the polynomial is prime.m3 - mn2 + m2n - n3
A. (m + n)(m - n) B. (m + n)2(m - n) C. prime D. (m - n)2(m + n)