Decide whether the given number is a solution to the equation preceding it.4p + 9p - 3 = 88; 7
A. Yes
B. No
Answer: A
You might also like to view...
Factor completely.20z2 + 3z - 9
A. (20z + 3)(z - 3) B. (4z + 3)(5z - 3) C. (20z + 1)(z - 9) D. (4z - 3)(5z + 3)
Find y in terms of x.
= 12(4x - 2)-3, curve passes through (0, 1)
A. y = - 6(4x - 2)-2 + 1.375
B. y = (4x - 2)-2 - 2.25
C. y = - 
(4x - 2)-2 + 1.375
D. y = - 
(4x - 2)-4 + 1.047
Decide whether or not the points are the vertices of a right triangle.Consider the three points A = (3, -2), B = (1, 0), C = (-3, -4). Determine whether the triangle ABC is a right triangle. Explain your reasoning.
A. The side lengths of triangle ABC are d(A, B) = 2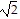
, d(A, C) = 2
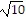, d(B, C) = 4.
[d(A, B)]2 + [d(B, C)]2 = (2
)2 + 42 = 8 + 16 = 24
[d(A, C)]2 = (2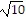
)2 = 40
Since [d(A, C)]2 ? [d(A, B)]2 + [d(B, C)]2 , triangle ABC is not a right triangle.
B. The side lengths of triangle ABC are d(A, B) = 2
, d(A, C) = 2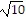
, d(B, C) = 4
.
[d(A, B)]2 + [d(B, C)]2 = (2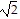
)2 + (4
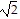)2 = 8 + 32 = 40
[d(A, C)]2 = (2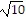
)2 = 40
Since [d(A, C)]2 = [d(A, B)]2 + [d(B, C)]2, triangle ABC is a right triangle.
C. The side lengths of triangle ABC are d(A, B) = 4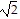
, d(A, C) = 2
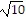, d(B, C) = 4.
[d(A, B)]2 + [d(B, C)]2 = (4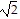
)2 + 42 = 32 + 16 = 48
[d(A, C)]2 = (2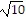
)2 = 40
Since [d(A, C)]2 ? [d(A, B)]2 + [d(B, C)]2, triangle ABC is not a right triangle.
D. The side lengths of triangle ABC are d(A, B) = 2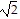
, d(A, C) = 4
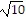, d(B, C) = 4
.
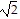[d(A, B)]2 + [d(B, C)]2 = (2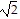
)2 + (4
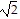)2 = 8 + 32 = 40
[d(A, C)]2 = (4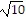
)2 = 160
Since [d(A, C)]2 ? [d(A, B)]2 + [d(B, C)]2, triangle ABC is not a right triangle.
Decide whether or not the ordered pair is a solution to the equation.x + 1 = 0; (-1, 8)
A. Yes B. No