Use the graph to evaluate the expression.a. (f ? g) (3)b. (g ? f) (0)c. (g ? g) (0)d. (f ? f) (-2)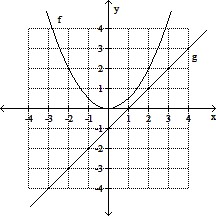
A. a. -2
b. -2
c. 1
d. 2
B. a. 3
b. 0
c. -1
d. -2
C. a. 2
b. -1
c. -2
d. 2
D. a. -1
b. 3
c. 0
d. -2
Answer: C
You might also like to view...
Solve the problem.If a person puts one penny in a piggy bank on the first day, two pennies in on the second day, three pennies in on the third day, and so forth, how much money will be in the bank after 60 days?
A. $36.60 B. $18.30 C. $9.15 D. $0.60
Solve the problem.The amount of time it takes a swimmer to swim a race is inversely proportional to the average speed of the swimmer. A swimmer finishes a race in 37.5 seconds with an average speed of 4 feet per second. Find the average speed of the swimmer if it takes him 50 seconds to finish the race.
A. 5 ft/sec B. 2 ft/sec C. 3 ft/sec D. 4 ft/sec
Solve the problem.A couple have bought a new house and are comparing quotes from two moving companies for moving their furniture. Company A charges $100 for the truck and $50 per hour for the movers. Company B charges $90 for the truck and $60 per hour for the movers. Create a cost equation for each company where y is the total cost and x is the number of hours of labor. Write a system of equations.
A. y = 100x + 50 y = 90x + 60 B. 50y = 100x 60y = 90x C. y = 50x + 100 y = 60x + 90 D. 50y = x + 100 60y = x + 90
Solve the problem.Ken is 6 feet tall and is walking away from a streetlight. The streetlight has its light bulb 14 feet above the ground, and Ken is walking at the rate of 4.2 feet per second. Find a function, d(t), which gives the distance Ken is from the streetlight in terms of time. Find a function,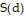 
, which gives the length of Ken's shadow in terms of d. Then find 
.
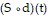A. (S ? d)(t) = 3.99t B. (S ? d)(t) = 3.15t C. (S ? d)(t) = 2.31t D. (S ? d)(t) = 7.1t