Complete the table and use the result to find the indicated limit.If f(x) = x2 + 8x - 2, find 
f(x). 
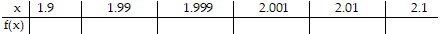
A.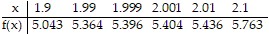 
; limit = 5.40
B. 
; limit = 17.70
C.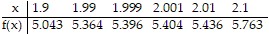 
; limit = ?
D.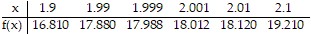 
; limit = 18.0
Answer: D
You might also like to view...
Solve the problem.Eye color (brown or blue) of a child is determined by which of two alleles (B for the dominant brown allele or b for the recessive blue allele) the child receives from each of its parents. A person is considered heterozygous for a trait if their two alleles are different than each other. If two heterozygous parents (Bb) have children, we expect 1 out of every 4 of those children to have blue eyes. How many blue-eyed children would we expect out of 860 children born to two heterozygous parents?
A. 172 blue-eyed children B. 645 blue-eyed children C. 430 blue-eyed children D. 215 blue-eyed children
Evaluate the improper integral or state that it is divergent.
A. 1 B. Divergent C. 0 D. -1
For the pair of functions, find the product function or functional value indicated.Find (fg)(x).f(x) = 6xg(x) = 4x - 4
A. (fg)(x) = 24x2 - 24x B. (fg)(x) = -24x2 - 24x C. (fg)(x) = 10x2 - 4x D. (fg)(x) = 24x - 24
Solve the problem.A dentist can sell his practice for $1,100,000 cash or for $250,000 plus $270,000 at the end of each year for 4 years. (a) Find the present value of the annuity that is offered if money is worth 8% compounded annually. (b) If she takes the $1,100,000, spends $250,000 of it, and invests the rest in a 4-year annuity at 8% compounded annually, what size annuity payment will she receive at the end of each year? (c) Which is better, taking the $250,000 and the annuity or taking the cash settlement?
A. (a) $894,274.25; (b) $256,632.68; (c) $250,000 plus the annuity has a larger present value than $1,100,000. She should take $250,000 plus the annuity. B. (a) $697,533.91; (b) $255,726.92; (c) $250,000 plus the annuity has a smaller present value than $1,100,000. She should take the $250,000 plus the annuity. She should take the cash settlement. C. (a) $992,644.41; (b) $253,613.48; (c) $250,000 plus the annuity has a larger present value than $1,100,000. She should take $250,000 plus the annuity. D. (a) $581,278.26; (b) $241,234.72; (c) $250,000 plus the annuity has a smaller present value than $1,100,000. She should take the cash settlement.