Find the domain of the function.
?
What will be an ideal response?
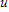and 
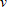
You might also like to view...
Answer the question.The graph below shows the level curves of a differentiable function f(x, y) (thin curves) as well as the constraint g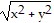(x, y) = 
- 
= 0 (thick circle). Using the concepts of the orthogonal gradient theorem and the method of Lagrange multipliers, estimate the coordinates corresponding to the constrained extrema of f(x,y).
A. (1.3, 0.7), (-1.3, 0.7), (-1.3,-0.7), (1.3,-0.7) B. (1.5, 0.2), (0.7, 1.3), (-1.5, 0.2), (-0.7, 1.3), (-1.5, -0.2), (-0.7, -1.3), (1.5, -0.2), (0.7, -1.3) C. (1.1, 1.1), (-1.1, 1.1), (-1.1,-1.1), (1.1,-1.1) D. (1.5, 0), (0, 1.5), (-1.5, 0), (0, -1.5)
Divide, if possible.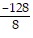
A. -26
B. - 
C. -16
D. 16
Find the prime factorization of the number. If the number is prime, state this.90
A. 2 ? 2 ? 3 ? 3 ? 5 B. 10 ? 3 ? 3 C. 2 ? 3 ? 5 D. 2 ? 3 ? 3 ? 5
Factor by factoring out the negative of the GCF.-3t2 + 12t + 15
A. -3t(t2 + 4t + 5) B. -3(t2 - 9t - 12) C. -3(t2 - 4t - 5) D. -3(t2 - 12t - 15)