Solve the polynomial equation. In order to obtain the first root, use synthetic division to test the possible rational roots. x3 + 8x2 - 18x + 20 = 0
A. {1 + i, 1 - i, -10}
B. {1 + i, 1 - i, 10i}
C. {-10, 10}
D. {1 + i, 1 - i, 10}
Answer: A
You might also like to view...
Find an explicit formula for an.8, 9, 10, 11, 12, . . .
A. an = n + 9 B. an = n + 7 C. an = n + 8 D. an = n - 8
Find the slope of the line (if it exists) and the y-intercept (if it exists).y = -1.8x - 5.4
A. Slope -5.4, y-intercept (0, -1.8) B. Slope -1.8, y-intercept (0, 5.4) C. Slope 1.8, y-intercept (0, -5.4) D. Slope -1.8, y-intercept (0, -5.4)
The table shows, for some particular year, a listing of several income levels and, for each level, the proportion of the population in the level and the probability that a person in that level bought a new car during the year. Given that one of the people who bought a new car during that year is randomly selected, find the probability that that person was in the indicated income category. Round your answer to the nearest hundredth.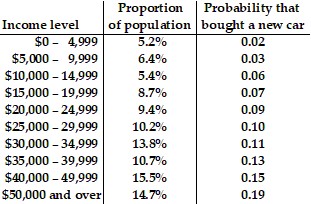 
$50,000 and over
A. 0.28 B. 0.24 C. 0.22 D. 0.25
For the equation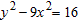 
, find the intercepts and the equations of the asymptotes.
A. intercepts: (3, 0), (-3, 0) asymptotes: y = -4x, y = 4x B. intercepts: (4, 0), (-4, 0) asymptotes: y = -3x, y = 3x C. intercepts: (4, 0), (-4, 0) asymptotes: x = -3y, x = 3y D. intercepts: (0, 4), (0, -4) asymptotes: x = -3y, x = 3y E. intercepts: (0, 4), (0, -4) asymptotes: y = -3x, y = 3x