Use the maximum/minimum finder on a graphing calculator to determine the approximate location of all local extrema.f(x) = x4 - 4x3- 53x2 - 86x - 80
A. Approximate local maximum at 1.032; approximate local minima at -3.255 and 7.098
B. Approximate local maximum at 1.027; approximate local minima at -3.126 and 7.188
C. Approximate local maximum at -0.944; approximate local minima at -3.192 and 7.136
D. Approximate local maximum at 1.029; approximate local minima at -3.282 and 7.138
Answer: C
You might also like to view...
Use Descartes' Rule of Signs to determine the possible number of positive real zeros and the possible number of negative real zeros for the function.P(x) = 5x20 - 7x14 - 6x11 + 6x2 - 5x
A. 3 positive; 2 negative B. 1 or 3 positive; 0 or 2 negative C. 0 or 2 positive; 0 or 2 negative D. 1 or 3 positive; 1 or 3 negative
Complete the square and write the equation in standard form. Then give the center and radius of the circle.x2 + y2 - 12x - 6y + 33 = 0
A. (x + 6)2 +(y + 3)2 = 12
(-6, -3), r = 2
B. (x - 6)2 +(y - 3)2 = 12
(-6, -3), r = 2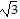
C. (x - 6)2 +(y - 3)2 = 12
(6, 3), r = 2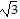
D. (x - 6)2 +(y - 3)2 = 12
(6, 3), r = 12
Find the exponential function that satisfies the given conditions.Initial population = 1129, doubling every 7 hours
A. P(t) = 7 ? 2t
B. P(t) = 1129 ?  t/7
t/7
C. P(t) = 1129 ? 2t/7
D. P(t) = 1129 ? 27t
Multiply using the FOIL method.(5x + 8)(5x + 7)
A. 25x2 + 75x + 56 B. 25x2 - 5x + 56 C. 25x2 + 56 D. 10x2 + 15